Here are two classic mistakes:
Whenever I see a mistake that recurs at all different levels, and with all different students, I wonder: what makes this mistake so attractive? What’s the misconception? And what can we do about it?
Say something smart in the comments, and then go check out this post from Fawn Nguyen.

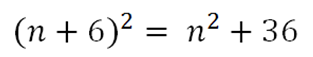
6 replies on “Classic Mistakes with Exponents”
I have to say that I don’t get why we talk about “the distributive property” of multiplication over addition, but not that of exponentiation over multiplication. If naming the distributive property is helpful in one circumstance, why wouldn’t it be helpful in the other? And if the reason we don’t discuss it w/r/t exponentiation is because it’s too abstract, then why do we think it’s helpful w/r/t multiplication?
These mistakes boil down to the fact that exponentiation distributes over multiplication, but not over addition. Whether this fact is true seems worth exploring with algebra students.
I’ve never heard of “the distributive property” of exponentiation over multiplication, but I’ve certainly heard my whole life the phrase “distributing the exponent” when referring to this rule:
$(ab)^n = a^n b^n$
I think the prior comments have essentially nailed it. The trouble is the “distributive property”.
We have that 2(3 + x) = 6 + 2x.
So why can’t we distribute from the other side? (3 + x)^2 = 3^2 + x^2?
Because it’s an exponent, we explain. You’re missing terms in the middle. Got it?
Good, because by the way, (3x)^2 = 3^2 x^2
Yeah, we can do that because we’re multiplying, not adding. Exponents just can’t distribute over ADDITION. They can distribute here – oh, by using addition. In the exponents.
So, comes the uncertain reply, 3x^2 is a multiplication, so I distribute. 3^2 x^2
We counter, actually, no brackets there, it’s just “3 x x”.
Honestly, I think this sort of thing needs the tile/area visual as backup – memorizing ‘rules’ can be problematic when things get so similar. Also, it probably doesn’t help that there’s a tendency to teach ‘perfect squares’ and ‘difference of squares’ at the same time, almost ensuring that the concepts will get jumbled together.
I have found that students have a hard time understanding (n + 6) as its own quantity. I agree that tiles/area can be very helpful. I have also found application problems to be a good tool to help students conceptualize that quantity. In a square with side lengths n + 6, it’s that whole quantity that gets multiplied to find the area.
I also ask my students to think about the order of operations. If we find a value for n, what is the first thing you would do?
In the first example: square n, then multiply that answer by 3. (so the 3 is never squared)
In the second: add 6, then square that answer.
I have students write it out. And 3n means 3 noses. n+6 means a nose and $6. Completely different.
But I do love that we are on mastery – you stay on this one project until you get it right! They are all helping each other, it’s okay if we make mistakes, we’re not hurrying.
[…] [Compare: http://mathmistakes.org/?p=396] […]