Category: Multiplying Polynomials

Noteworthy:

• The kids have a ton of confidence, even in the stuff that they haven’t formally studied in class yet.  (For this survey, Questions 1-3 had been covered formally, and Questions 4-5 had not.) To my mind, this continues to reaffirm that the most annoying mistakes aren’t the distortion of instruction; they’re the failure of instruction to override preconceptions.
• Kids like to say that 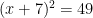, and teachers like to say that this is due to overuse of the Distributive Property. That might be true, but those teachers also have to recognize that kids said that 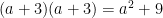with almost the same verve and frequency. It’s hard to blame exponents or notation for that mistake, right? So where does this intuition come from?
• A couple of kids included a 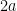term in Q4 and a term in Q5. I find this interesting, but I’m not exactly sure what its significance is. Is the temptation to add and when the binomials are in the same visual position that they are for addition problems?

The idea that kids walk into our classes with these intuitions is, I think, counter to the way that most math teachers talk and think about these mistakes. I think that realizing that these mistakes are the result of deep intuitions about how math should be is important. I also think thinking about where these intuitions come from is important, because maybe we can avoid setting them in earlier years.

I hope that some of you will give this survey to your students who haven’t yet received instruction on how to multiply polynomials. The original survey can be found here.

You’ll disagree with me in the comments, right? I’m counting on you all…