Complex Division

Thanks to John Burk for the submission. He writes, “I know this student wouldn’t simplify (6+2x)/(3-x) this way.”

So: if the student wouldn’t simplify (6+2x)/(3-x) this way, then how did the student end up messing it up for (6+2i)/(3-i)?

crazedmummy

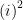How would you simplify $\dfrac {\Gamma \left( 6+2x\right) } {3-x}$ ? It’s not the same, because multiplying by complex conjugate doesn’t get rid of the x in the denominator. I think $\left( i\right) ^{2}$ is a different fish from $\left( x\right) ^{2}$
Also, the multiplication of the two binomials is a math mistake in its own right. Two for one, thanks!
crazedmummy

sorry, no idea why that peculiar symbol is there, please ignore it. Just a LaTex learner.
mpershan

Via Colleen, who commented on the picture on a different page: “This student assumes that (a+b)/(c+d) = a/b+b/d. We could show him that this doesn’t work with numbers?”
abshores

I agree with Colleen that we could use specific numbers to show (a+b)/(c+d) != a/c + b/d. However, that never sticks. How do we permanently knock this misunderstanding out of students minds?