We shouldn’t have to reconstruct what was going through the students mind! Students should be required to explain their work as they go and docked points even for correct computations when they don’t.
It appears the left column is an abandoned line of thought and this student was too lazy to erase or cross it out.
It also appears that the student views the dot product as adding corresponding components, rather than multiplying them and then adding the resulting products. Teaching her the correct procedure for computing the dot product would probably get the student close to a perfect solution. Well, I’d think so if she hadn’t written that disturbing first column of work.
A larger issue for this student that won’t be rectified easily — the student was performing zero meta-cognition when she thought it would be possible to solve 3+4=0 and two other linear equations for t. She was in rote procedure mode. But yes, from the premise that the three equations at the top of the right column hold, anything can be made to follow from completely valid work. I don’t blame the student for being confident in her algebra (which only has a minor sign error). I hope she wasn’t confident in those initial premises, but I bet it didn’t occur to her to question them.
So, bear in mind I’m just an idiot who spends her time on “add 3 to both sides.” “of the equal sign.”
But if the dot product is zero,doesn’t it mean that
Which I don’t see anywhere. Then and so t=-4
Or am I wrong? Do tell, since i lost my mind in pre-algebra…
The student seems to think that adding each part of the vector has something to do with it. I don’t see a dot multiplication anywhere. I would ask the student what has to be zero.
2 replies on “Perpendicular Vectors”
We shouldn’t have to reconstruct what was going through the students mind! Students should be required to explain their work as they go and docked points even for correct computations when they don’t.
It appears the left column is an abandoned line of thought and this student was too lazy to erase or cross it out.
It also appears that the student views the dot product as adding corresponding components, rather than multiplying them and then adding the resulting products. Teaching her the correct procedure for computing the dot product would probably get the student close to a perfect solution. Well, I’d think so if she hadn’t written that disturbing first column of work.
A larger issue for this student that won’t be rectified easily — the student was performing zero meta-cognition when she thought it would be possible to solve 3+4=0 and two other linear equations for t. She was in rote procedure mode. But yes, from the premise that the three equations at the top of the right column hold, anything can be made to follow from completely valid work. I don’t blame the student for being confident in her algebra (which only has a minor sign error). I hope she wasn’t confident in those initial premises, but I bet it didn’t occur to her to question them.
So, bear in mind I’m just an idiot who spends her time on “add 3 to both sides.” “of the equal sign.”
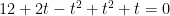 and
and  so t=-4
so t=-4
But if the dot product is zero,doesn’t it mean that
Which I don’t see anywhere. Then
Or am I wrong? Do tell, since i lost my mind in pre-algebra…
The student seems to think that adding each part of the vector has something to do with it. I don’t see a dot multiplication anywhere. I would ask the student what has to be zero.