6 replies on “The Distributive Property of Absolute Value”
I have problem with my students trying to distribute with the absolute value. I have always talked about making sure that the absolute value is isolated or (by itself) before you go on to simplify any further. However, some students go into auto-pilot and distribute anyway.
Any suggestions?
Maybe the | bars look too much like parentheses. What if you rewrote it as 3 abs(x+8) = 3, emphasizing that abs is a function and why you can’t distribute over functions? Or maybe that will be even more confusing to someone without my programming skills?
While we’re on the topic, it’s quite unfortunate that x*f(y+z) looks so similar to x*(y+z). Hmm… Maybe we could say there’s an invisible identity function in front of the second set of parentheses?
The student who “authored” this mistake is just learning to speak English. She has been in this country for 3 months. She is very good with algorithms. But I think the notation was an easy mistake for her to make, confusing the absolute value bars with parentheses. But yet at the end of the problem she tied in something about absolute value into her solution.
In class, the and I use Google translation app to “talk” back and forth. She sits at my computer and types in any directions she is not sure of. I wonder if we should be using “Find the absolute value of 2x if x = -6” instead of “evaluate |2x| for x = -6. Yet if she had not learned “absolute value” back in her former country, that would probably have little meaning. 🙂
But we’re pretty hypocritical as math teachers. Why would a student distribute anyway? If we use the “undo”method, you would always divide both sides by three. (In my school, it’s so we can avoid using fractions.)
The difficulty does not appear to me to be the distribution, because you can indeed distribute across the absolute value sign. The problem appears to be that then the student has not chosen the two possible values for inside the absolute value. produces and gives us which I think are the solutions.
So I would be asking “what choices of numbers can you put in between the absolute value signs to get 3?” This sort of crunches on “what equal means,” one of my pet projects.
You could probably make an intersting lesson out of “distributing” into absolute values. Does it matter if you “distribute” a variable instead of a number?
As others have pointed out, isolating the absolute value is the standard way to go, and probably the simplest and least confusing method. I believe a lot of students struggle with this because they can’t get their focus away from “x” as the variable. In this problem you really want to think of |x + 8| as the variable. Solve for |x + 8| first, then worry about x. It is this additional layer of abstraction that causes a lot of issues for students. Same issue comes up with composing functions, solving sq root, log, trig equations, etc.
Thanks, Mr B. In calculus,we substitute for terms, so we could do and then do What a good idea! Then we wouldn’t be suddenly putting that in.
6 replies on “The Distributive Property of Absolute Value”
I have problem with my students trying to distribute with the absolute value. I have always talked about making sure that the absolute value is isolated or (by itself) before you go on to simplify any further. However, some students go into auto-pilot and distribute anyway.
Any suggestions?
Maybe the | bars look too much like parentheses. What if you rewrote it as 3 abs(x+8) = 3, emphasizing that abs is a function and why you can’t distribute over functions? Or maybe that will be even more confusing to someone without my programming skills?
While we’re on the topic, it’s quite unfortunate that x*f(y+z) looks so similar to x*(y+z). Hmm… Maybe we could say there’s an invisible identity function in front of the second set of parentheses?
The student who “authored” this mistake is just learning to speak English. She has been in this country for 3 months. She is very good with algorithms. But I think the notation was an easy mistake for her to make, confusing the absolute value bars with parentheses. But yet at the end of the problem she tied in something about absolute value into her solution.
In class, the and I use Google translation app to “talk” back and forth. She sits at my computer and types in any directions she is not sure of. I wonder if we should be using “Find the absolute value of 2x if x = -6” instead of “evaluate |2x| for x = -6. Yet if she had not learned “absolute value” back in her former country, that would probably have little meaning. 🙂
But we’re pretty hypocritical as math teachers. Why would a student distribute anyway? If we use the “undo”method, you would always divide both sides by three. (In my school, it’s so we can avoid using fractions.)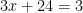 produces
produces  and
and  gives us
gives us 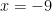 which I think are the solutions.
which I think are the solutions.
The difficulty does not appear to me to be the distribution, because you can indeed distribute across the absolute value sign. The problem appears to be that then the student has not chosen the two possible values for inside the absolute value.
So I would be asking “what choices of numbers can you put in between the absolute value signs to get 3?” This sort of crunches on “what equal means,” one of my pet projects.
You could probably make an intersting lesson out of “distributing” into absolute values. Does it matter if you “distribute” a variable instead of a number?
As others have pointed out, isolating the absolute value is the standard way to go, and probably the simplest and least confusing method. I believe a lot of students struggle with this because they can’t get their focus away from “x” as the variable. In this problem you really want to think of |x + 8| as the variable. Solve for |x + 8| first, then worry about x. It is this additional layer of abstraction that causes a lot of issues for students. Same issue comes up with composing functions, solving sq root, log, trig equations, etc.
Thanks, Mr B. In calculus,we substitute for terms, so we could do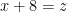 and then do
and then do 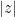 What a good idea! Then we wouldn’t be suddenly putting that in.
What a good idea! Then we wouldn’t be suddenly putting that in.